88 Future Value, Single Amount

Since the number of periods (n or t) is one, FV=PV(1+i), where i is the interest rate.

The amount of time between the present and future is called the number of periods. A period is a general block of time. Usually, a period is one year. The number of periods can be represented as either t or n.

Suppose you’re making an investment, such as depositing your money in a bank. If you plan on leaving the money there for one year, you’re making a single-period investment. Any investment for more than one year is called a multi-period investment .

Let’s go through an example of a single-period investment. As you know, if you know three of the following four values, you can solve for the fourth:

1. Present Value (PV)
2. Future Value (FV)
3. Interest Rate (i or r) [Note: for all formulas, express interest in it’s decimal form, not as a whole number. 7% is.07, 12% is.12, and so on. ]
4. Number of Periods (t or n)

In a single-period, there is only one formula you need to know: FV=PV(1+i). The full formulas, which we will be addressing later, are as follows:

Compound interest:

$\text{FV} = \text{PV} \cdot (1+\text{i})^\text{t}$

For example, suppose you deposit $100 into a bank account that pays 3% interest. What is the balance in your account after one year?

In this case, your PV is $100 and your interest is 3%. You want to know the value of your investment in the future, so you’re solving for FV. Since this is a single-period investment, t (or n) is 1. Plugging the numbers into the formula, you get FV=100(1+.03) so FV=100(1.03) so FV=103. Your balance will be $103 in one year.

Multi-Period Investment

Multi-period investments take place over more than one period (usually multiple years). They can either accrue simple or compound interest.

There are two primary ways of determining how much an investment will be worth in the future if the time frame is more than one period.

The first concept of accruing (or earning) interest is called “simple interest. ” Simple interest means that you earn interest only on the principal. Your total balance will go up each period, because you earn interest each period, but the interest is paid only on the amount you originally borrowed/deposited. Simple interest is expressed through the formula in.

Suppose you make a deposit of $100 in the bank and earn 5% interest per year. After one year, you earn 5% interest, or $5, bringing your total balance to $105. One more year passes, and it’s time to accrue more interest. Since simple interest is paid only on your principal ($100), you earn 5% of $100, not 5% of $105. That means you earn another $5 in the second year, and will earn $5 for every year of the investment. In simple interest, you earn interest based on the original deposit amount, not the account balance.

The second way of accruing interest is called “compound interest. ” In this case, interest is paid at the end of each period based on the balance in the account. In simple interest, it is only how much the principal is that matters. In compound interest, it is what the balance is that matters. Compound interest is named as such because the interest compounds: Interest is paid on interest. The formula for compound interest is.

Suppose you make the same $100 deposit into a bank account that pays 5%, but this time, the interest is compounded. After the first year, you will again have $105. At the end of the second year, you also earn 5%, but it’s 5% of your balance, or $105. You earn $5.25 in interest in the second year, bringing your balance to $110.25. In the third year, you earn interest of 5% of your balance, or $110.25. You earn $5.51 in interest bringing your total to $115.76.

Compare compound interest to simple interest. Simple interest earns you 5% of your principal each year, or $5 a year. Your balance will go up linearly each year. Compound interest earns you $5 in the first year, $5.25 in the second, a little more in the third, and so on. Your balance will go up exponentially.

Simple interest is rarely used compared to compound interest, but it’s good to know both types.

Calculating Future Value

The Future Value can be calculated by knowing the present value, interest rate, and number of periods, and plugging them into an equation.

When calculating a future value (FV), you are calculating how much a given amount of money today will be worth some time in the future. In order to calculate the FV, the other three variables (present value, interest rate, and number of periods) must be known. Recall that the interest rate is represented by either r or i, and the number of periods is represented by either t or n. It is also important to remember that the interest rate and the periods must be in the same units. That is, if the interest rate is 5% per year, one period is one year. However, if the interest rate is 5% per month, t or n must reflect the number of periods in terms of months.

Approaches to Calculating Future Value

Calculating FV is a matter of identifying PV, i (or r), and t (or n), and then plugging them into the compound or simple interest formula.

The method of calculating future value for a single amount is relatively straightforward; it’s just a matter of plugging numbers into an equation. The tough part is correctly identifying what information needs to be plugged in.

As previously discussed, there are four things that you need to know in order to find the FV:

1. How does the interest accrue? Is it simple or compounding interest?
2. Present Value
3. Interest Rate
4. Number of periods

Let’s take one complex problem as an example:

On June 1, 2014, you will take out a $5000 loan for 8-years. The loan accrues interest at a rate of 3% per quarter. On January 1, 2015, you will take out another $5000, eight-year loan, with this one accruing 5% interest per year. The loan accrues interest on the principal only. What is the total future value of your loans on December 31, 2017?

First, the question is really two questions: What is the value of the first loan in 2017, and what is the value of the second in 2017? Once both values are found, simply add them together.

Let’s talk about the first loan first. The present value is $5,000 on June 1, 2014. It is possible to find the value of the loan today, and then find it’s value in 2017, but since the value is the same in 2017, it’s okay to just imagine it is 2014 today. Next, we need to identify the interest rate. The problem says it’s 3% per quarter, or 3% every three months. Since the problem doesn’t say otherwise, we assume that the interest on this loan is compounded. That means we will use the formula in. Finally, we need to identify the number of periods. There are two and a half years between the inception of the loan and when we need the FV. But recall that the interest rate and periods must be in the same units. That means that the interest must either be converted to % per year, or one period must be one quarter. Let’s take one period to be one quarter. That means there are 10 periods. Please note that we don’t really care when the loan ends in this problem–we only care about the value of the loan on December 31, 2017.

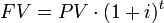
Next, we simply plug the numbers into. PV=5000, i=.03, and t=10. That gives us a FV of $6,719.58.

Now let’s find the value of the second loan at December 31, 2017. Again, PV=$5000, but this time, pretend it is January 1, 2015. This time, the interest is 5% per year and it is explicitly stated to be simple interest. That means we use the formula in. January 31, 2017 is exactly two years from the January 1, 2015 and since the interest is measured per year, we can set t=2 years.

When we plug all of those numbers into, we find that FV=$5,500.00

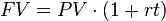
Since the problem asks for the total FV of the loans, we add $6,719.58 to $5,500.00, and get a total value of $12,219.58